Portfolio Construction Part 2 – The Matrix Revolution

In my previous post I looked at the sort of factors you need to consider when deciding what level of diversification is right for you. In this post I’m going to look at a way of improving how you weight those assets within your portfolio.

There is actually a mathematical method for determining what proportion of one's portfolio you should allocate to certain investment opportunity. It's called Kelly criterion and is based on the expected return and the odds of receiving that return.

https://en.wikipedia.org/wiki/Kelly_criterion

This can be shown mathematically to maximise one’s return over the long term. Unfortunately, although mathematically optimal, applying the Kelly criterion to portfolio construction has a few issues:

1. It is based on a consecutive series of events which you can determine the odds and payoff for. It doesn’t generalize well to portfolio management where you are choosing between competing concurrent investment opportunities.
2. Probably the biggest issue with applying the Kelly criterion is the level of draw-downs you can expect. These would be enough to end most investing careers if realised. For this reason even practitioners that use the Kelly criterion explicitly use a fraction of the amount that the Kelly criterion suggests.
3. The final issue is that the Kelly criterion assumes that one can accurately assess the odds of success and the payoffs. If you get the odds wrong or have a large level of uncertainty (as is likely when investing in individual stocks) there is a big variation in what proportions you should apply.

So rather than give a specific mathematical formula I suggest the following simple framework based on similar principles to help you allocate assets or test your portfolio in a logical way.

The first principle is that you should hold more of stocks that you believe to have the greatest upside.

It is perfectly valid to have a portfolio where you believe a set of stocks as a group to be fundamentally undervalued but you are not able to determine any difference in potential between them. This would be akin to a simple quant strategy e.g. buying the cheapest decile of stocks. In this case it makes sense to equal weight your portfolio. However for the rest of this post I’m going to assume that there is an ability to at least roughly assess the potential upside of a company and that you want to make the most of that potential upside.

The second principle is that you would like to bear the least amount of risk in achieving that upside.

Now when I say risk here I do not mean, as academics often do, just volatility or the co-variance of price movements. These can matter to investors. Particularly professional money managers who bear career or asset flow risk during periods of low returns. Or the private investor who bears ‘volatitility risk’ by explaining to a spouse why they are going to Blackpool on holiday this year not Barbados! However these are not the only forms of risk. What I am talking about here is an honest assessment potential downside if things go wrong or the risk of a permanent loss of capital. Forms of risk on top of volatility one may want to account for are:

Financing risk – Companies with high levels of debt will be less able to weather a period of poor trading. A heavily loss making company may struggle to raise extra capital.

Management risk – Management with a history of poor capital allocation may not act in the best interests of shareholders.

Product risk – Companies with one product or a few products serving only one market are more exposed to the performance of that product or market. Typically, but not exclusively, this makes smaller companies riskier.

Liquidity risk – The risk of not being able to sell near the published price when you want to. Typically, but not exclusively, this makes smaller companies riskier.

Commodity risk – Companies without a sustainable competitive advantage to control pricing are more likely to be exposed to commodity, economic growth, inflation or obsolescence factors.

Correlation Risk – I don’t mean the mathematical co-variance of stocks but the common exposure they have to factors that are out of your control. For example if you already have an oil explorer in your portfolio adding a second one should be considered higher risk (all other things being equal) than adding an equally undervalued oil consumer such as a plastics manufacturer.

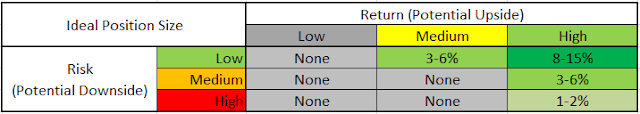
By assessing the stocks in your portfolio & watch-list on these principles you can then use the following matrix to determine portfolio weightings:

• If you find investments that are both low risk and high potential return then you should invest heavily in these.

• For low risk investments with a good return potential or slightly riskier stocks with an excellent expected return you can hold a reasonable amount.

• If you find very high return stocks that are also risky and a conservative assessment of the expected return is positive you should invest. It’s just that you should limit yourself to small positions in these types of opportunities. If they come off the returns will be high even from modest positions.

• Finally why would you choose to hold any investment if it’s going to generate a low return whatever the risk level?

The precise percentage values will vary depending on your chose diversification level (see Part 1) and the availability of good investment ideas however these sorts of levels tend to work for me with a typical 25 long positions:

If this seems simple to you it is because it is. And if we all invested our capital fresh every day we would probably naturally gravitate towards something like this. However typical portfolios evolve over time. Capital is added or removed at different times. Stock prices change and with them our portfolio weightings. Because we all suffer from anchoring effects and ownership bias our portfolios can quickly vary from the ideal without us taking action. Therefore the real power of applying this framework is being able to assess your current portfolio against it. Too often we buy a high risk position that goes up significantly and becomes a large position. Typically this then has same or increased downside risk but reduced upside as its valuation becomes stretched yet it has become one of our biggest positions. Often we hold on without selling as it becomes a small position weight again! Conversely when we really have found a low risk investment with a very high return we may fail to put enough into it to really take advantage.

One of the common objections to rebalancing portfolios is ‘I like to run my winners and cut my losers.’ However this only works as a strategy because share prices exhibit medium term positive serial correlation i.e. they have momentum. And you’ll notice that I’ve specifically not mentioned valuation in my assessment of potential upside. As a value investor my primary method for assessing potential upside is valuation metrics however yours may be something different like technicals. Whatever your method the matrix still gives a good logical framework for asset allocation. There is good academic for momentum i.e. that prices that have risen for 6-12 months tend to keep on rising therefore it can be right to include the price momentum in determining the potential upside. Just that one should be consciously doing this and if that is the primary component of upside that remains one needs to sell when momentum fades. Like all other forms of excess return it requires disciplined execution to capture effectively.

For advanced investors you can expand the matrix to include shorting high risk stocks that have low expected returns. And the high risk but very high return potential of options strategies.

But like all complex investing strategies they should be used cautiously with small diversified positions and only after getting the basics right.

So in summary, good position sizing can seem less exciting than choosing your next winning stock however it is equally important to acheiving consistently good returns. It makes sense to apply the principles that you want to maximize your upside and minimize your downside to all your portfolio positions. I believe that creating your own risk-reward matrix, and consciously assessing your portfolio against this, will provide a framework that will keep you in line with these over time and lead to better long term returns.

In the last part of this trilogy I will look at some simple rules that can help avoid other behavioral biases creeping into our portfolios.